Search
astrophysics (85)biophysics (18)chemistry (23)electric field (71)electric current (75)gravitational field (81)hydromechanics (146)nuclear physics (44)oscillations (57)quantum physics (31)magnetic field (43)mathematics (89)mechanics of a point mass (298)gas mechanics (87)mechanics of rigid bodies (221)molecular physics (71)geometrical optics (78)wave optics (65)other (167)relativistic physics (37)statistical physics (21)thermodynamics (154)wave mechanics (51)
mechanics of a point mass
(2 points)3. Series 25. Year - 1. Watt's regulator
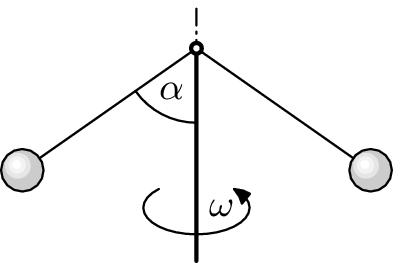
On attached picture you can see a system consisting of two heavy balls attached to a joint using two rigid rods. Assume that the motion of the balls is constrained to a certain vertical plane. The whole system starts to rotate around a vertical axis passing through the joint. How does the angle $α$ depend on the angular velocity of the system $ω?$
Aleš po cestě do otevřených dveří.
(4 points)3. Series 25. Year - 4. cutting down a tree
There can be many problems when cutting down a tree. Imagine a rod attached to an unstretchable rope placed on a pulley. Two workers are standing under the tree to make sure the rod does not fall into a pool. The rod falls down a distance $h$ before the rope is tightened. Under certain conditions the workers that hold the rope can be puled so high that they hit the pulley. Give conditions under which cutting of this branch is safe.
Hint: First consider two masses on ice connected by an unstretchable rope and having distinct velocities.
Michal odpaloval rakety.
(8 points)3. Series 25. Year - E. bouncing of a ball
Roll a very flexible ball perpendicularly against a wall. When it bounces off the wall it jumps. How does the distance of the point where the ball hits the ground depend on the initial velocity of the ball? You can also investigate dependence on other parameters.
Note You can find some useful information in the text on our web.% (http://fykos.cz/rocnik25/3-e_std-text.pdf).
(4 points)2. Series 25. Year - 4. ball on a train
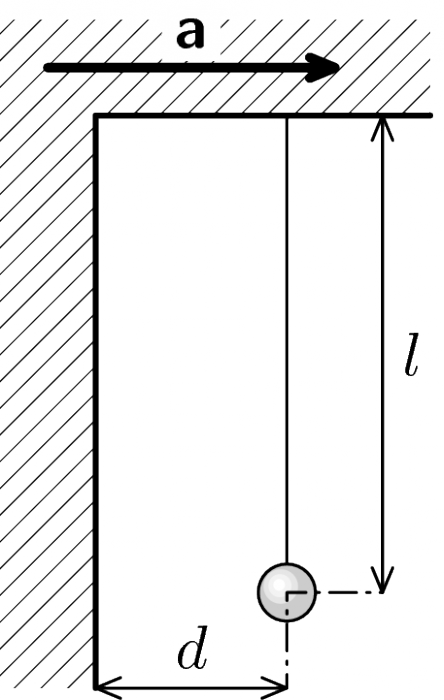
Small weight of mass m is suspended from the ceiling of a train on a string of length $l$. The weight is hanging a distance $d$ from a wall (see picture). Suppose the train starts to accelerate in the direction perpendicular to the wall. Find a condition on the acceleration such that the weight eventually touches the wall. How long does it take before this happens? Assume that $d$ is less than $ l/5$$.
(4 points)2. Series 25. Year - 5. hair
A hair with a lot of curls can be approximated by a spring (in other words it can be described by the exact same parameters – radius, inclination and material constants). How much longer is the hair if it hangs vertically in a gravitational field compared to the case that it lies horizontally on a table? Assume that the inclination is very small.
(5 points)2. Series 25. Year - P. elevator
Suppose that you are in an elevator when the rope that holds it breaks. Can you save yourself from dying by jumping right before the elevator hits the ground? What is the maximum speed of the falling elevator such that you could save yourself this way? You will need to look up or estimate the maximum impact velocity that a human can survive.
(2 points)1. Series 25. Year - 2. struggling swimmer
A man wants to swim across a river which flows at a speed of 2 km/h. He is able to swim at a speed of 1 km/h. What is the optimal trajectory and direction he should take so that his trip is the least exhausting? Where and at what time will he reach the other bank? How would the situation change if his aim was the shortest possible trajectory? The width of the river is $d=10\;\mathrm{m}$.
Petr
(4 points)1. Series 25. Year - 4. drrrrr
A small conductive ball of negligible size is bouncing between two charged plates of opposite polarities. What is the frequency of the resulting periodic motion of the ball? Voltage between the plates is $U$. When the ball touches a charged plate it charges to a charge of magnitude $Q$ whose polarity is the same as polarity of the plate. The ratio of kinetic energies of the ball before and after an impact is $k$.
Bonus: Does the output power of this resistor correspond to the energy losses during impacts?
Jáchym
4. Series 24. Year - 2. To the Sun
Karel has decided to throw his notes into the Sun. Help: him calculate the necessary initial velocity of his notebook so that it reaches the Sun. You can neglect the frictional froces acting on his notebook and you can also assume that the trajectory of the Earth around the Sun is circular. You know the masses of the Earth and the Sun as well as the distance of the Earth from the Sun.
Karel
1. Series 24. Year - E. shot put
We all know that in vacuum if we throw an object under a certain angle and with some initial speed the distance it will travel is independent upon the mass of the object. If we drop the vacuum assumption, the problem is not so simple. Experimentally find how does the distance of the point where the object hits the ground depends on its mass and shape. Make theoretical predictions (numerical calculations are allowed) and compare them with your findings.